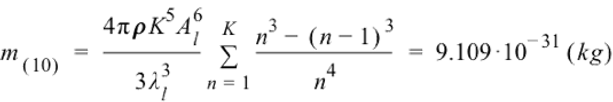Description

The rest energy of an object is based on its mass, otherwise known as mass-energy equivalence – Albert Einstein’s famous E=mc2 equation. Although Einstein wasn’t the first to relate mass and energy, he was the first to propose the correct relationship in 1905 as a part of relativity.  The equation relates energy (E) equal to mass (m) times the speed of light (c) squared.  However, why is it related to the square of the velocity at which light travels the universe?

 

 

 

 

 

 

 

 

 

 

 

 

 

 

In energy wave theory, mass is the sum of a particle’s standing waves of longitudinal waves. A standing wave consists of an in-wave and an out-wave, both traveling at the speed of light (c). Therefore, mass is the representation of standing waves without consideration of the wave speed. Energy is the representation of standing waves with consideration of the in-wave and out-wave speeds (c2).  A standing wave has no net propagation of energy.  It is stored energy, which is what is measured in particles as either energy or mass.

The equation represents a particle’s energy at rest (velocity = 0).  When a particle is in motion, especially at relativistic speeds, it gains energy/mass.  This is described in the next page.

 

 

 

 

 

 

 

Derivation – Classical Constants

There is no derivation available for the mass-energy equivalence equation using classical constants. The wave constant derivation is preferred as it describes mass as standing waves, and its energy inclusive of the speed of the in-wave (c) and out-wave (c).

 

 

 

Derivation – Wave Constants

The wave constant derivation begins with the Energy Wave Equation from the wave constant form. The energy for a particle is based on its number of wave centers (K). Energy and mass equations are simply a difference between the speed of light squared. This derivation includes descriptions that are addressed in the Particles section, such as the volume and radius assumptions, and definitions of wave centers and shells. It is highly suggested that the reader visit the Particles section first to understand this derivation. Further details are in the Key Physics Equations and Experiments paper.

 

 

​

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Proof

Particle energies were calculated in Particles, including the electron which found to have 10 wave centers (K). The calculation of electron mass is below. It matches in both numerical value and units.  Units are kg.